Head pressure refers to the force exerted by a fluid, such as water or gas, at a specific point in a closed system in relation to a reference point.
In this article, we will explore the concepts of hydrostatic and dynamic head pressure, the different methods for measuring head pressure, and the factors that influence head pressure.
Definition of Head Pressure
Head pressure, in the context of fluid mechanics, pertains to the pressure exerted by a fluid due to the weight of the fluid above a certain reference point. It is an important concept in engineering, especially when dealing with the design and analysis of fluid systems.
Hydrostatic Head Pressure

Elevate Your Engineering With Excel
Advance in Excel with engineering-focused training that equips you with the skills to streamline projects and accelerate your career.
Hydrostatic head pressure is the pressure exerted by a fluid at rest. The magnitude of this pressure is directly proportional to the fluid’s density, the acceleration due to gravity, and the height of the fluid column above the point of measurement. This can be expressed by the formula:
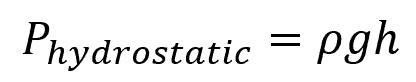
Where:
- Phydrostatic = hydrostatic head pressure [Pa]
- ρ = density of the fluid [kg/m3]
- g = gravitational acceleration [9.81 m/s2]
- h = height of the fluid above the reference point [m]
This equation implies that the hydrostatic head pressure is independent of the shape of the container and relies solely on the height of the fluid column.
Dynamic Head Pressure
Dynamic head pressure, also known as velocity head, relates to the pressure of moving fluids. It is derived from the fluid’s velocity and density as follows:
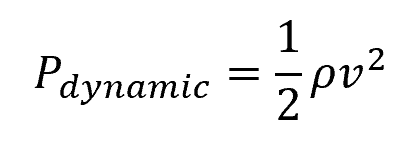
Where:
- Pdynamic = dynamic head pressure [Pa]
- v = velocity of the fluid [m/s]
Dynamic head pressure is typically considered in systems where fluid is in motion, such as pipelines and pumps. It represents the kinetic energy per unit volume of a fluid due to its motion.
Head Pressure Measurement
In the field of fluid mechanics, head pressure measurement is a critical process, and several instruments are designed for this purpose. These instruments convert the physical property of head pressure into a readable value.
Differential pressure transmitters are commonly used for measuring the pressure differential between two points in a system. They can quantify the head pressure in terms of the height of a fluid column, providing an indirect measurement of head pressure.
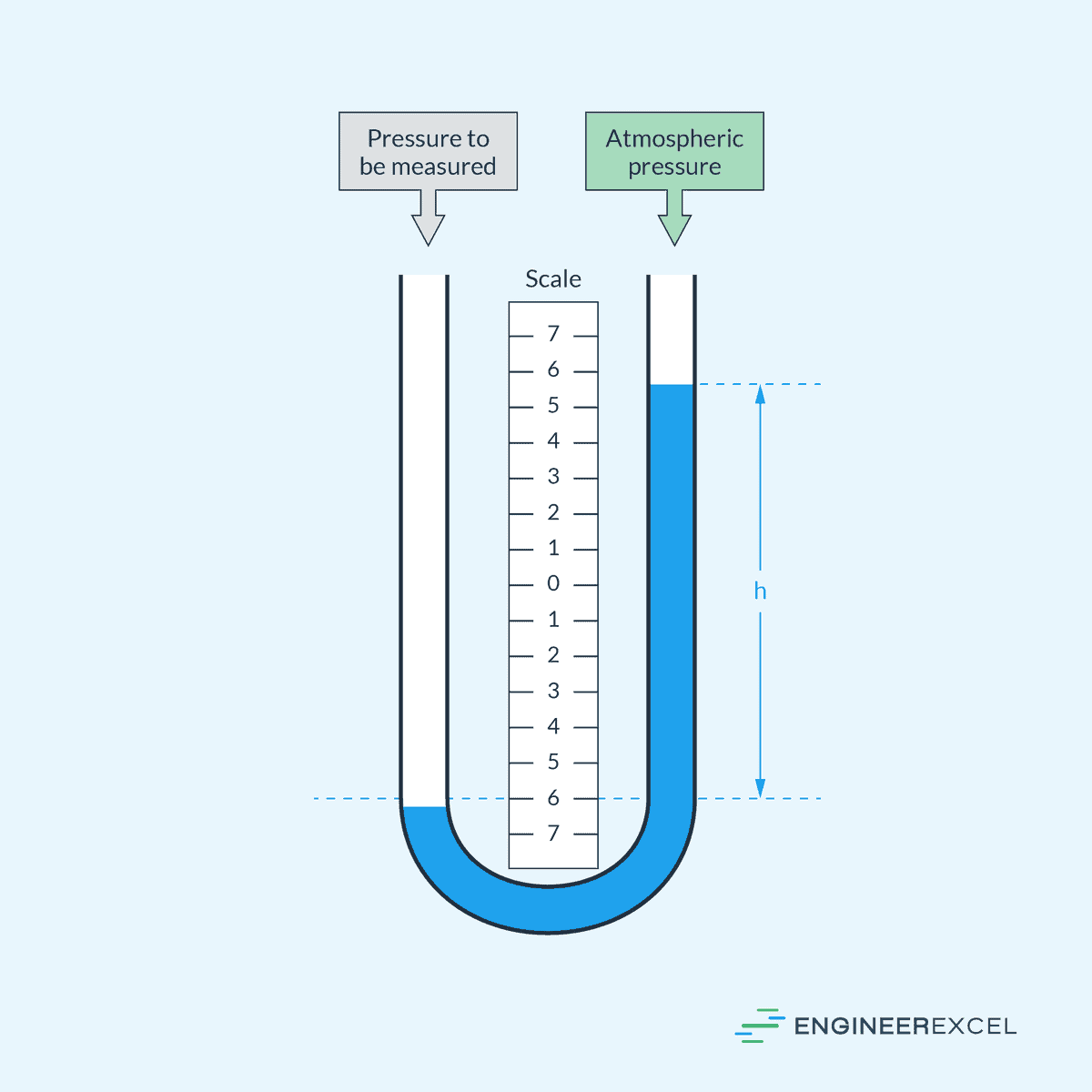
Manometers, another type, utilize columns of fluid in U-shaped tubes and can be simple and accurate for small scale applications. Their operation is based on the physical principle that the head pressure is proportional to the fluid column height difference.
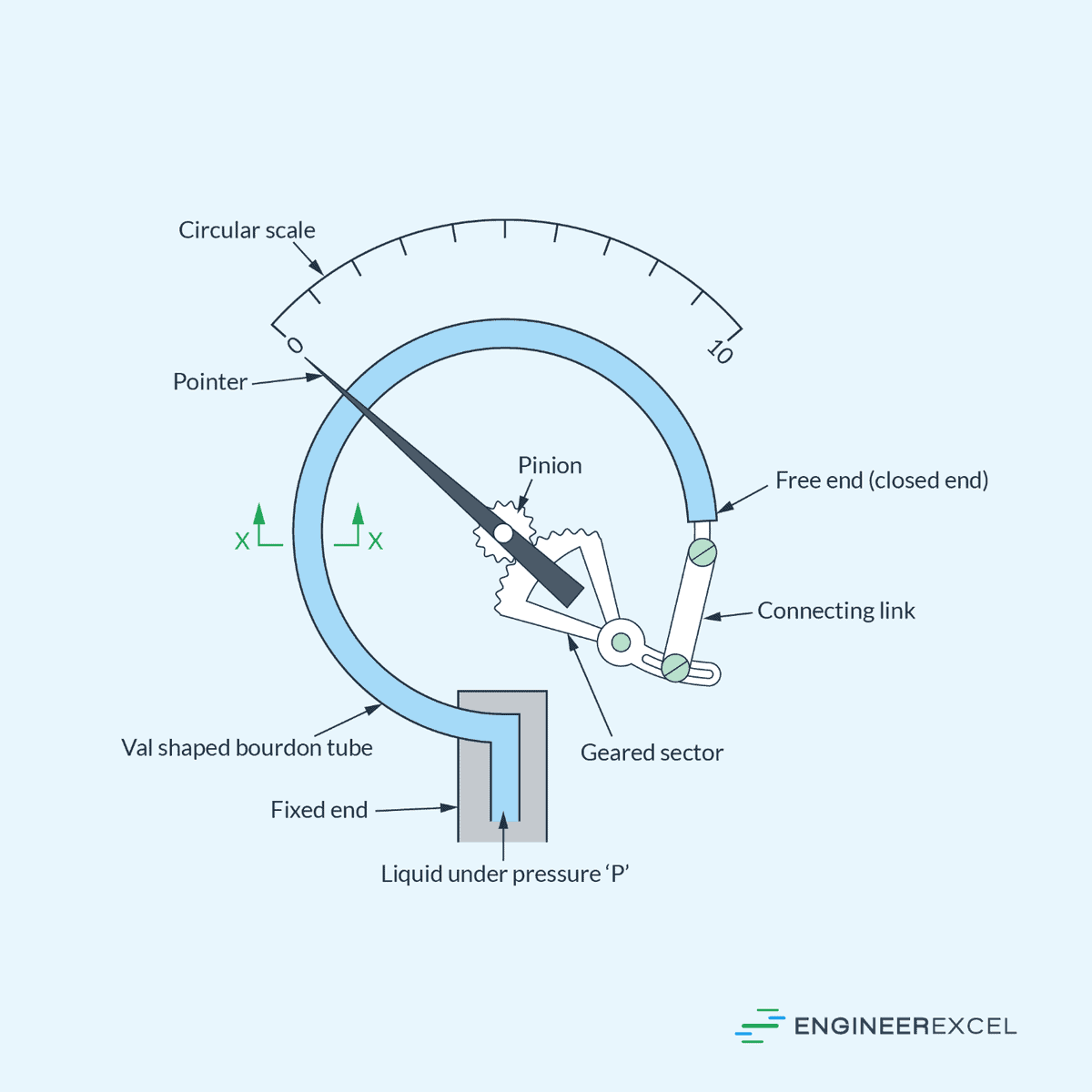
Pressure gauges are mechanical devices that provide a direct reading, usually via a dial. The head pressure causes a mechanical deflection in the gauge, which is translated into a pressure reading. These are robust instruments suitable for a wide range of industrial applications.
Some applications may also use ultrasonic sensors. These devices calculate pressure by sending an ultrasonic pulse through a medium and measuring the time it takes to return. Sensors are chosen based on the specific application and required accuracy levels.
For precision measurements, especially in laboratory conditions, digital pressure transducers are used. They offer high accuracy and can interface with computer systems for monitoring and data logging. Care must be taken to select an instrument with the appropriate range and sensitivity for the specific head pressure measurement task at hand.
Factors Affecting Head Pressure
Several factors influence head pressure, and understanding them is essential for accurate fluid system design.
Fluid Density
The density of a fluid is directly proportional to its head pressure. Fluids with higher density exert greater head pressure at a given height due to the increased weight per unit volume. For example, pumping mercury, which has a higher density than water, will result in a higher head pressure for the same vertical distance.
Pump Speed
In pump systems, the speed at which a pump operates also affects the head pressure. Faster pump speeds generate higher pressure due to the increased force they impart on the fluid. Care must be taken when adjusting the speed to avoid damages caused by excessive pressure within the system.
Pipe Diameter
The diameter of the pipes in a system has an inverse relationship with head pressure. With other conditions constant, narrower pipes result in higher fluid velocity, which can cause an increase in dynamic head pressure. On the other hand, wider pipes lower the velocity and, consequently, the dynamic head pressure.
Calculating the optimal pipe diameter is critical to maintaining efficient and safe operating conditions.
Challenges and Considerations
Pressure Loss
Pressure loss occurs when the head pressure decreases as fluid flows through a system due to friction and other resistive forces. Engineers must account for this loss when designing systems by calculating the friction factor through Darcy-Weisbach or Hazen-Williams equations, dependent on the fluid type and pipe material.
Cavitation
Cavitation is a phenomenon where vapor bubbles form in a liquid at low-pressure areas and collapse in higher-pressure areas, potentially causing damage to equipment. To prevent cavitation, it is vital to maintain the pressure throughout the system above the fluid’s vapor pressure and to carefully monitor the Net Positive Suction Head (NPSH).
Pump Selection and Placement
Selecting and placing pumps correctly in a system is important in managing head pressure. Pumps must be chosen based on their performance curves to meet the required flow rate and head pressure. Placement is critical; for instance, pumps should be installed close to the fluid source to minimize suction side pressure loss and avoid cavitation.
