True strain is a measure of deformation representing the natural logarithm of the ratio of the current length to the original length of a material undergoing deformation. It provides a more accurate description of strain in large deformations than engineering strain, which is calculated as the change in length divided by the original length. In this article, we delve into the true strain equation, its derivation, measurement, and practical applications in engineering.
What is True Strain
In engineering, strain is a measure of the deformation of a material relative to its original dimensions. A common way to quantify strain is by dividing the change in the length of a specimen by its original length:
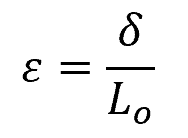
Where:
- ε = engineering strain [unitless]
- δ = total elongation of the specimen [m]
- Lo = original length of the specimen [m]

Elevate Your Engineering With Excel
Advance in Excel with engineering-focused training that equips you with the skills to streamline projects and accelerate your career.
The strain calculated in the above formula is known as nominal or engineering strain, which assumes a constant strain throughout the material. However, in reality, this assumption is not always accurate. To provide a more precise description of the material’s strain behavior, true strain is used.
The true strain, also referred to as logarithmic strain, is the natural logarithm of the ratio of the current length to the original length of a material undergoing deformation:
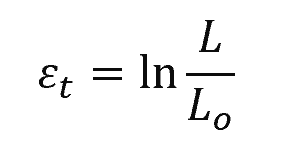
Where:
- εt = true strain [unitless]
- L = current length of the specimen [m]
This measure takes into account the continuous changes in the material’s geometry during the deformation process. In contrast to engineering strain, true strain offers a more accurate assessment of deformation for large strains as it considers the instantaneous dimensions of the specimen.
Derivation of True Strain Equation
The derivation of the true strain equation is based on incremental deformation as the material is stretched, which accumulatively gives the net strain. To begin with, consider an infinitesimal segment of a material under tension that elongates by a small increment of length dL from its initial length L.
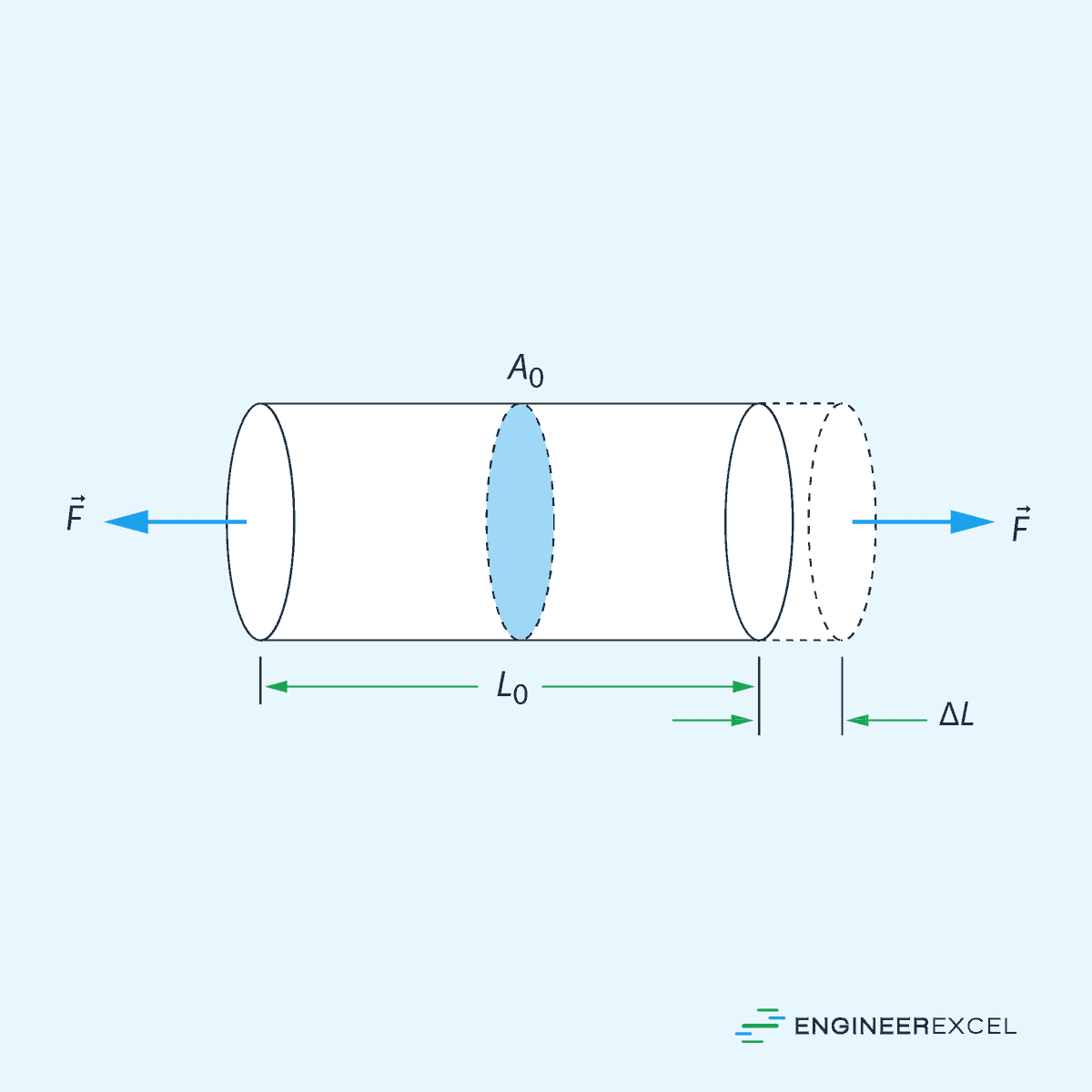
The infinitesimal strain increment, dε, can thus be expressed as dL/L. This ratio represents a small change in length relative to the current length of the material segment at any given instant during the deformation process.
Hence, for an infinitesimal increment, true strain is given by:
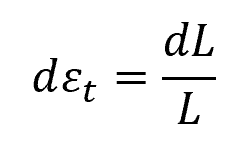
Integration of the strain increments over the entire deformation process is necessary to obtain the total true strain. By integrating the strain increment expression from the initial length Lo to the current length L, one obtains the true strain as follows:
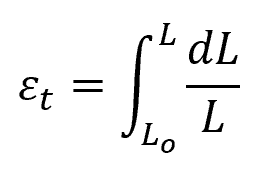
This integral, when evaluated, yields the logarithmic strain equation:
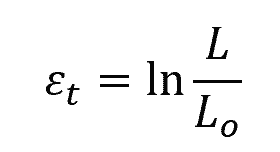
True Stress-Strain Diagram
True stress and true strain provide a more accurate depiction of how materials behave when they undergo plastic deformation, compared to traditional engineering stress and strain. The differences between these concepts are demonstrated in the diagram below, showing the typical stress-strain curve for a ductile material under tension.
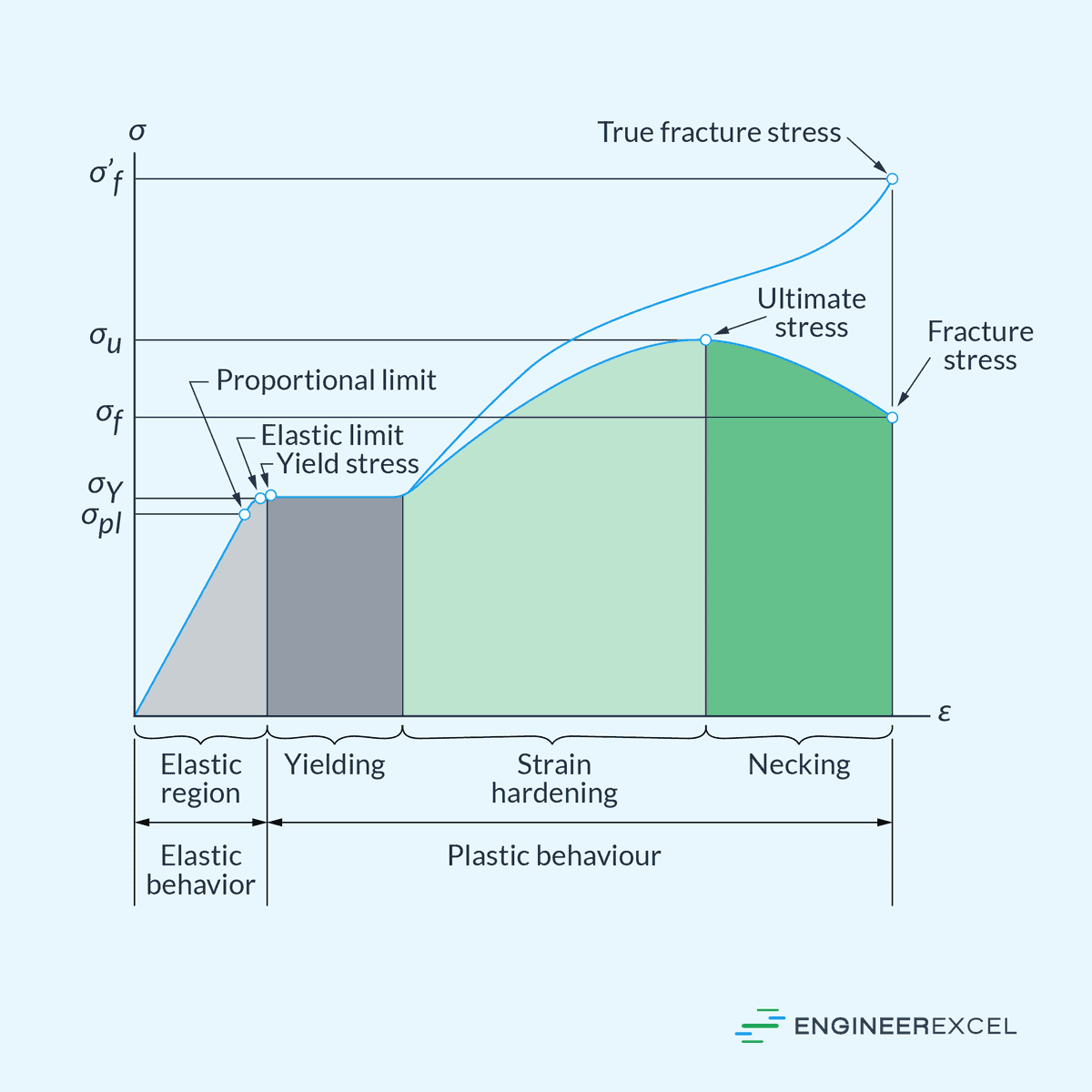
In the case of true stress, the calculation of stress is based on the instantaneous cross-sectional area, as opposed to the original area used in engineering stress. True strain, on the other hand, accounts for the incremental deformations that occur throughout the material’s entire history of deformation.
During the elastic region of deformation, the behavior of the material is both linear and reversible, with little to no noticeable difference between the engineering and true stress-strain curves. However, after surpassing the yield point, the material’s deformation becomes non-linear and irreversible. It is beyond this yield point that the disparities between the engineering and true values start to become pronounced.
As strain-hardening progresses, the material’s strength increases until necking becomes dominant, eventually resulting in fracture.
Measuring True Strain
In practice, true strain can be measured during a tensile test by tracking the change in length of the specimen as it is being deformed and calculating the natural logarithm of the ratio of this deformed length to the original length. This can be done using various methods, such as extensometers, digital image correlation, or other strain measurement techniques.
Extensometry
Extensometry is a technique that involves the use of devices called extensometers, which are attached to the specimen undergoing testing. They measure the change in length between two points on the specimen’s surface.
Dial gauge extensometers are common for simple applications, whereas electronic extensometers offer high precision and can provide digital readouts for analysis. The selection of an extensometer depends on the required measurement range and desired accuracy.
Digital Image Correlation
Digital Image Correlation (DIC) is a non-contact optical method that analyzes the changes in a digital image of the specimen’s surface before and after deformation. It involves applying a random speckle pattern on the specimen surface and tracking the movement of the pattern through successive images taken during the test. This technique is known for its ability to measure strain over a large surface area with high accuracy and detail, making it particularly useful for complex or non-uniform deformations.
Practical Applications
Material Testing
In material testing, the true strain equation facilitates the precise calculation of elongation in tensile tests. Engineers employ it to assess material ductility, which is important when determining a material’s suitability for specific applications that require flexibility or elongation before failure. The data obtained from such tests, represented as stress-strain curves, are essential in understanding a material’s mechanical properties and behavior.
Plastic Deformation Analysis
When materials exceed their elastic limit and enter the plastic deformation phase, the true strain equation becomes essential for analyzing permanent deformation. In processes like metal forming or plastic extrusion, this analysis is critical to ensuring that the formed parts will meet design specifications and maintain their integrity under operational conditions. Predicting the limit of ductility is also useful to prevent catastrophic failures during manufacturing or in-service application.
