When fluid flows through a PVC pipe, frictional forces between the fluid and the pipe walls cause a pressure drop along the pipe’s length. In fluid mechanics, this drop in pressure is characterized by a dimensionless parameter known as the friction factor.

This article tackles friction factor for PVC pipes and its calculation methods.
Friction Factor For PVC Pipe
A PVC pipe is a lightweight and durable type of plastic pipe made from Polyvinyl Chloride (PVC). It finds extensive use in waterlines, waste pipes, drainpipes, gutters, and downspouts due to its affordability, chemical resistance, and ease of joining. Compared to traditional galvanized steel piping, PVC is lighter, making it more convenient to handle.
One of the significant advantages of PVC pipes is their smooth inner lining, which helps prevent sediment buildup and blockages. As shown in the table below, PVC only has an absolute roughness of 0.000084 in. or 0.00021 cm— the lowest among the listed pipe materials.

Elevate Your Engineering With Excel
Advance in Excel with engineering-focused training that equips you with the skills to streamline projects and accelerate your career.
This smoothness also results in lower friction losses compared to other types of pipes. As a result, PVC pipes can efficiently transport fluids over long distances with minimal pressure loss.
To calculate the pressure loss through a PVC pipe, the Darcy-Weisbach equation is commonly used:
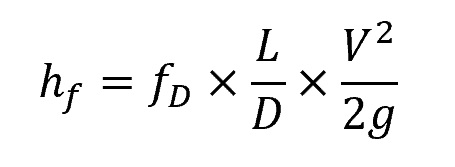
Where:
- hf = head loss due to friction [m]
- fD = Darcy friction factor [unitless]
- L = length of the pipe [m]
- D = hydraulic diameter of the pipe [m]
- V = mean fluid velocity [m/s]
- g = gravitational constant [9.81 m/s2]
The Darcy friction factor is a dimensionless parameter that quantifies the flow resistance of a fluid in a pipe. The higher the friction factor, the higher the head loss, assuming the same flow conditions.
In general, the value of the friction factor is a function of the Reynolds number, the shape of the conduit, and the roughness of the inner surface of the pipe. Because PVC pipes are relatively smooth, they also have some of the lowest friction factors compared to other types of pipe materials. For turbulent flow, the friction factor for PVC pipe can range from 0.005 to 0.04, depending on the Reynolds number.
Calculating Friction Factor For PVC Pipe
The formula used for calculating friction factor depends on the flow regime. For laminar flow, the friction factor is simply equal to:
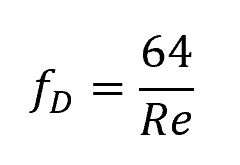
Where:
- Re = Reynolds number [unitless]
As shown in the equation above, the friction factor for laminar flow is solely determined by the Reynolds number. In this case, the friction factor decreases inversely with the Reynolds number.
However, in most practical applications, flow is actually turbulent in nature. For turbulent flow, the friction factor is generally influenced by both the Reynolds number and the roughness of the pipe surface. However, since PVC pipes are considered smooth, it is usually safe to ignore the roughness of the pipe wall.
For smooth-walled pipes like PVC, the following equation, developed by Prandtl, can be used to obtain the friction factor:
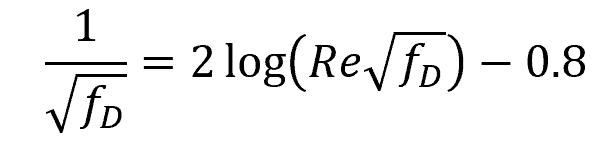
Using the above formula, the friction factor for smooth-walled PVC pipe has been calculated at different Reynolds numbers and tabulated below:
Notice that the value of the friction factor for smooth-walled pipes only drops by a factor of 5 over a 10,000-fold increase in Reynolds number.
Since the equation above is implicit in nature, it can be cumbersome to solve for friction factor if Reynolds number is known. Fortunately, there are alternative approximations from which friction factor for smooth-walled pipes can be computed explicitly from Reynolds number, as shown in the following equations:
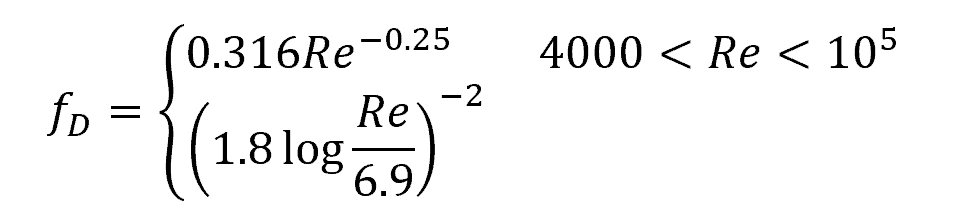
Although the equations above give good approximations of friction factor for smooth-walled PVC pipes, it is still more accurate to account for pipe roughness, especially if roughness data is readily available. In this case, the Colebrook equation can be used to calculate for friction factor:
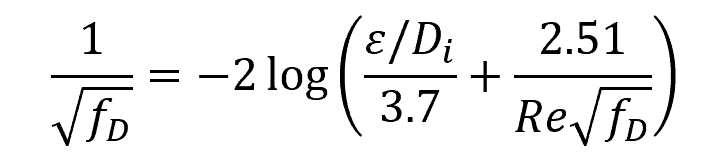
Where:
- ε = absolute roughness of the inner pipe surface [cm or in]
- Di = internal diameter of the PVC pipe [cm or in]
Alternatively, friction factor can be estimated using the Moody chart, shown below:
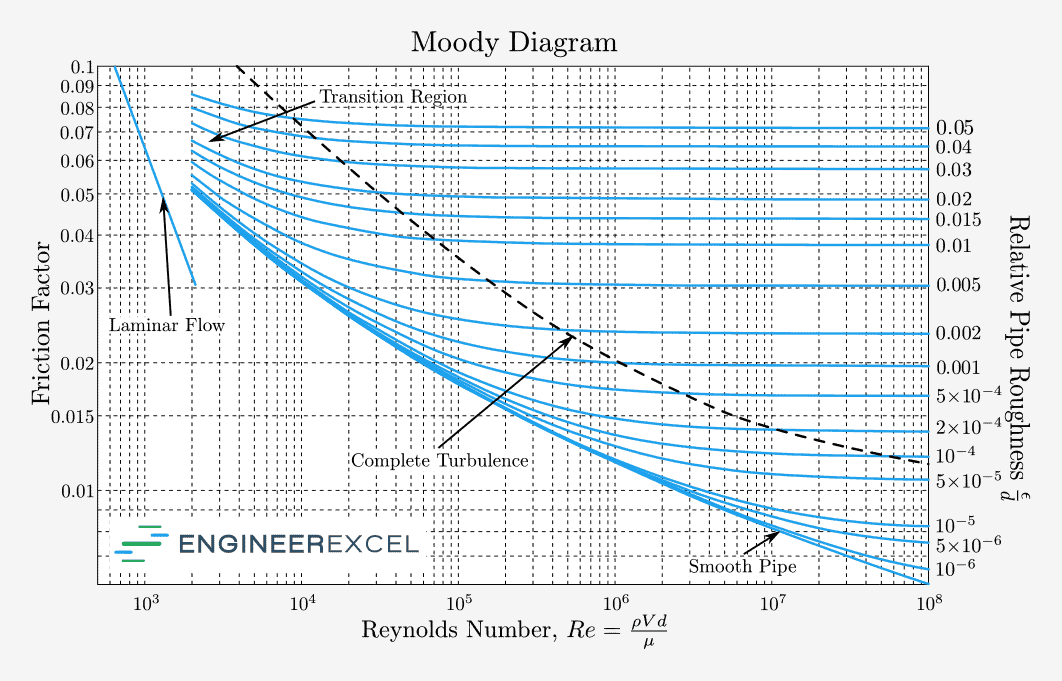
The Moody chart is accurate to ±15 percent and can be used for both circular and noncircular PVC pipe flows.
Notice that for very high Reynolds numbers, the curve transitions into a horizontal line; that is, the friction factor becomes independent of Reynolds number. In this case, the flow is said to be fully rough and the friction factor becomes solely dependent on relative roughness, as shown in the following formula:
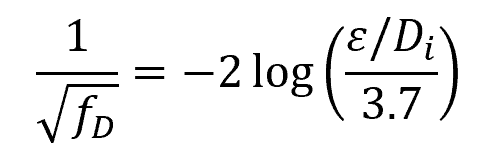
Roughness Of PVC Pipes
As shown in the above equations, the surface roughness of a PVC pipe significantly impacts the friction factor of the flow. Hence, it is crucial to use the correct roughness value when doing friction loss calculations.
For PVC pipes, the typical absolute roughness is around 0.000084 inches or 0.00021 centimeters, which is a safe assumption for most practical applications. However, it is important to note that different types of PVC materials, such as PVC-U, PVC-O, and PVC-M, may have varying roughness values. For highly sensitive applications, it may be necessary to measure pipe roughness using sensitive profiling instruments to detect nanometer-level height deviations.
Additionally, the roughness of a PVC pipe can be influenced by the manufacturing process and the installation environment. For example, pipes exposed to sunlight or chemicals might have higher roughness compared to those that aren’t.
While thinner PVC pipes generally have excellent inner surfaces, problems like waviness, spider lines, sink marks, burning lines, and black spots can arise as the thickness and diameter increase. All these factors impact the roughness value and, consequently, the friction factor of the PVC pipe flow.
