In fluid mechanics, pressure refers to the force exerted by a fluid per unit area, typically perpendicular to the surface it acts on. Head, on the other hand, represents the potential energy of a fluid due to its elevation above a reference point, often used to quantify the energy or pressure in a fluid system.

In this article, we will explore the relationship between head and pressure, their calculation, the different types of head, head losses in fluid systems, and the practical applications of these concepts in engineering.
Fundamentals Of Head And Pressure
The key concepts of head and pressure are fundamental in understanding fluid mechanics and systems involving fluid flow. These terms denote different but related aspects of how fluids behave and interact with their surroundings.
In fluid mechanics, head refers to the specific energy per unit weight of fluid due to its elevation above a reference level and the fluid’s pressure and velocity. It is commonly measured in meters (m) or feet (ft). Essentially, head represents the potential energy that a fluid possesses, which can be converted into work or kinetic energy.
Elevate Your Engineering With Excel
Advance in Excel with engineering-focused training that equips you with the skills to streamline projects and accelerate your career.
Pressure, on the other hand, is the force exerted by a fluid per unit area and is typically measured in Pascals (Pa) or pounds per square inch (psi). It manifests in fluids at rest and operationally quantifies the force the fluid exerts against the walls of its container or any surface it comes in contact with.
Head and Pressure Calculation
The relationship between head and pressure is intrinsic and can be mathematically related by the equation:
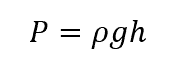
Where:
- P = pressure [Pa]
- ρ = fluid density [kg/m3]
- g = acceleration due to gravity [9.81 m/s2]
- h = head [m]
This equation illustrates that pressure can be understood as a measure of the fluid head – the height of a fluid column producing a specific pressure at the bottom due to gravity.
Types Of Head
In fluid dynamics, understanding the different types of head is important for analyzing fluid flow. Aside from pressure head, the other types of head include elevation head and velocity head, each accounting for specific energy forms within a hydraulic system.
Pressure Head
Pressure head is the height of a liquid column that would develop due to the fluid’s pressure. In technical terms, it is the internal energy of a fluid due to the pressure exerted on its container’s walls. This head is expressed in terms of the vertical height of a column of fluid that pressure could support and is calculated by dividing the fluid pressure by the product of fluid density and gravitational acceleration:
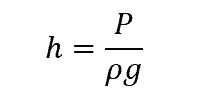
Elevation Head
Elevation head represents the potential energy of a fluid due to its elevation above a reference level. It is quantified by the vertical distance from this reference point to the fluid surface and is typically measured in meters (m). For example, water at a height of 10 meters has an elevation head of 10 m.
Velocity Head
Velocity head accounts for the kinetic energy of a moving fluid and is defined by the height that the fluid would reach if all its kinetic energy were converted into potential energy. The calculation is:
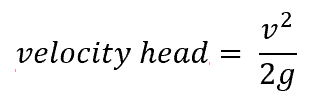
Where:
- v = fluid velocity [m/s]
The velocity head is significant in systems where fluid velocities are high, as in pipelines or open channels.
Head Losses In Fluid Systems
Head losses in fluid systems are fundamental to understanding fluid flow dynamics. Engineers differentiate between major losses associated with the length of the conduit and minor losses due to fittings, valves, and other devices.
Major losses are quantified by the Darcy-Weisbach equation:
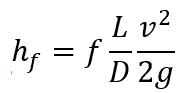
Where:
- hf = major head loss [m]
- f = friction factor [unitless]
- L = conduit length [m]
- D = hydraulic diameter [m]
The friction factor is a dimensionless quantity that characterizes the resistance to flow in a pipe or duct due to internal friction. Its value generally depends on the Reynolds number, geometry of the conduit, and surface roughness.
Minor losses, on the other hand, can be expressed as:
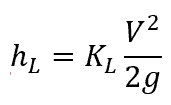
Where:
- hL = minor head loss due to a system component[m]
- KL = loss coefficient of the component [unitless]
The loss coefficient of a component depends on its geometry and material and can be obtained from experiments or empirical data. It is important to consider these losses for accurate prediction of fluid behavior in a conduit.
Applications In Engineering
Understanding the concept of head and pressure is important in the design and analysis of systems involving fluid flow.
Piping Network Analysis
A critical application of head and pressure in engineering is in the design and analysis of piping networks.

Accurate modeling of pressure losses due to friction, changes in elevation, and fittings is necessary to simulate the performance of a piping system under various operating conditions. This involves calculating the minimum pressure needed at different points to maintain adequate flow rates and designing pump and piping layouts that are economically and technically efficient.
Pressure Vessels
Pressure vessels are designed to operate safely at a predetermined pressure and temperature. Hence, it is important to perform stress analysis of materials when designing pressure vessels to prevent structural failure under operational stresses.
Regulations often require that vessels undergo pressure testing and inspections to ensure their reliability and safety over time. Understanding the distribution of stress caused by internal pressure helps in selecting appropriate materials and designing vessels that comply with safety codes.
